参考答案：A

解析：

解法1：

=sin²x+csc²x+cos²x+sec²x

=1+1+cot²x+1+tan²x

=3+cot²x+tan²x

因为tan²x+cot²x≥2|tanx|·|cotx|=2，

故当|tanx|=|cotx|，即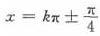(k∈Z)时，tan²x+cot²x取最小值2，即cot²x+tan²x≥2，

故所求函数y∈[5，+∞)，故正确答案为A。

解法2：

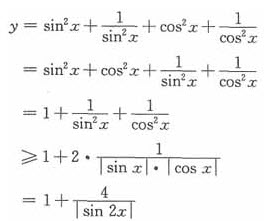
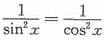当|sin2x|=1，(k∈Z)，即时，|sin2x|取最大值，这时正好有，则有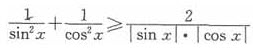的等号成立，故这时y取最小值1+4=5，故所求函数y∈[5，+∞)，故正确答案为A。

解法3：

当sin²2x=1，

故所求函数y∈[5，+∞)，

故正确答案为A。